全文HTML
-
长期以来,关于学生高层次数学认知能力的研究和培养一直是国际数学教育研究关注的焦点。如由美国匹兹堡大学Stein教授主持的QUASAR计划就明确指出,数学教学应培养学生思维、推理、问题解决以及数学交流的能力,而非简单的记忆与重复[1]。在我国,自21世纪初开始的新一轮数学课程改革中,一个极为重要的课程理念就是变革学生的数学学习方式,强调学生数学学习过程中的实验、猜测、推理和验证等高层次数学认知活动[2]。然而在实际教学中,学生高层次的数学认知能力却似乎没得到应有的发展。由顾泠沅领导的青浦实验小组分别在1991年和2007对初中二年级学生进行了测试和比较,其结果发现我国学生在中低层次认知水平上(如计算与概念等记忆层面的理解)获得长足的进步,而其高层次认知水平(探究性理解水平,如分析、解决问题的能力)的表现却几乎没有改进[3]。尤其是对于复杂的非常规问题的解决,几乎所有的学生都只能达到初级水平(如用简单的规则、公式与概念来计算一些简单的问题)而没有办法达到更高水平(如关联水平和高级抽象水平)[4]。有关中美学生问题解决能力的研究也发现中国学生仅在常规数学问题解决方面具有优势,但在复杂且具有创造性的开放任务、问题提出以及非常规问题的解决等方面,其表现比美国学生差[5]。
诚然,影响和制约学生数学认知水平提高的因素较多。但毫无疑问的是,学生所接触的数学任务是影响学生数学认知水平和数学思维发展的核心因素[6]。不同的数学任务需要学生进行不同认知层次的数学活动。因此,要培养学生高层次的数学认知能力,就有必要让学生更多地进行更高认知层次的数学任务[6]。长期以来,教材都是学生数学任务最为重要和直接的来源,其所包含的例题和习题都是学生获得数学概念、掌握数学定理、发展数学能力的重要载体[7],数学教材例题、习题中具体内容所包含的认知机会将更为直接地影响学生的数学能力和水平的发展。
近年来,关于数学教材内容的认知机会逐渐受到国际数学教育研究者的关注。国外有实证研究表明,如果学生所用教材中含有更多需要学生进行高层次数学认知活动的任务,学生的数学成绩将会更高[8]。但在国内,目前还鲜有研究从认知机会视角对我国数学教材进行系统检视。特别是没有研究比较我国数学教材与其他国家(特别是在国际数学学业成就比较中体现出较高水平的国家,如新加坡)数学教材中的认知机会。基于此,本文以中国和新加坡初中二年级数学教材为研究对象,系统比较两国初中数学教材中例题和习题所提供的认知机会,以期为我国后续教材建设和教学提供借鉴和参考。
-
本文选择中国、新加坡两国初中数学教材进行对比分析。依据教材使用的广泛性、对相应国家课程标准理念的体现以及教师使用时间三项指标进行对比,选取的两国教材具体信息如表 1所示。
-
例题及习题根据其在教材中所处位置和作用的不同,分为不同的模块。不同教材对模块的编排也有区别。本文统计的人教版教材例题和习题包括:例题、练习、习题和复习题;新数学教材统计的例题和习题有:“工作示例(worked example)”“课堂练习(practise now)”“习题(exercise)”“复习题(review rxercise)”“挑战自我(challenge yourself)”和“修正复习(revision exercise)”。若一题中含若干个小题,就根据题目中小题个数统计;若一题有连续多问,但这几问并未拆分形如(1)(2)(3)这样的形式,就当作一个题目统计。
-
数学教材提供的不同学习机会已经被证明会影响学生在认知领域的成就[8]。本文着重关注数学教材例题和习题所提供的认知领域的学习机会,即数学教材的认知机会。为描述数学任务中的认知机会,当前研究者主要从一个特定任务的“理解”层次来进行考量[9-10]。由此,对于分析教材的认知机会而言,就可以通过考查学生完成教材中的每项任务所需的理解水平、类型和理解复杂性来实现。
为了将学生的理解外显化,Perkins和Blythe从学生“表现”视角对理解进行了探索[11]。他们指出理解某个知识就是能够通过寻找证据和例子、归纳、应用、类比以及用新的方式表达主题等表现来展示对知识的理解。换句话说,理解是建立在学生展示各种表现的能力之上的,这些表现揭示了学生对该主题的理解。在此基础上,他们提出了“为理解而教”(Teaching for Understanding)的框架[11]。随后,Harpaz对该框架进行了进一步的研究和改进,提出了“理解表现”(Understanding Performance,UP)框架[12-13](详情如表 2所示)。
在改进的理解表现框架中,理解水平被分为4级,其中0级无需理解,也无认知要求;1、2、3级要求理解且水平逐级递增。1、2、3级每级均包含6种不同的理解表现,共18种理解表现(见表 2),不同理解表现对应不同思维方式。本研究借鉴该“理解表现”框架,用以分析和比较中新两国教材例题与习题涉及的思维类型、水平和复杂度,从而了解两套教材例题、习题的认知机会的异同。
对照UP框架,以人教版教材中的一道习题为例,进行具体编码。例如:一个等腰三角形的一边长为6cm,周长为20cm,求其他两边的长。学生要解决这道题,就需要辨别出已知边既可作为“底”,也可作为“腰”,同时还需要检查此两种情况是否都能围成三角形,故编码为“诠释”。
-
本研究采用定性分析和定量分析相结合的方法对中国、新加坡两国初中数学教材例题和习题数量、18种理解表现占比、认知复杂性、认知水平等方面进行分析, 对比两国初中二年级教材中的认知机会。具体方法为:依照UP框架对题目编码,鉴于一道题可能涉及一种理解表现,也可能多种,涉及的理解表现则赋值为1,其余赋值为0,若一道题目反复多次使用同一种理解表现仍赋值为1。
编码完成后,进一步分析了18种理解表现的占比。理解表现占比指每种理解表现出现的次数占总题数的百分比。占比越高,表明教材对该理解能力越重视。
随后,统计分析了各个问题的认知复杂性。认知复杂性指具体一道题目所用到的不同理解表现的数量。一道题目有时无需任何理解,只凭死记硬背的知识即可解答,而有时需要通过多种理解方可成功解决。由此,在解答题目的过程中,所用到的不同理解表现的数量就决定了一个题目的认知复杂性,故将题目认知复杂性定义为题中涉及的理解表现数量,并依次分为0、1、2、3、>3五级。
最后,对各个题目的认知水平进行了分析。认知水平指题目涉及的理解表现所处水平级数的最大值。记忆的理解水平为0,其他18种理解表现按理解水平被分为1、2、3级。若一个题目只涉及一种表现,认知水平则以该表现所处的水平级数赋值。若一个题目涉及多种表现,则以所处水平级数的最大值赋值。
同时,为了保证编码的可靠性,降低个人主观因素造成的误差,两名数学教育专业的研究生首先就编码框架讨论交流,统一认识,随后随机选取部分章节进行独立编码,编码一致性达93.21%。最后对差异部分重新协商,再予以确定,最终一致性达到99.28%,其可信度较高。编码和赋值后用EXCEL收集和整理数据。
一. 教材的选取
二. 研究内容
三. 比较框架及编码
四. 研究过程
-
将至少涉及一种理解表现的题目称为理解型题目,而不需任何理解表现就能完成的题目归属为记忆型题目。中国、新加坡两国初中数学教材例题和习题数量及记忆型题目与理解型题目的占比情况如表 3所示。
在总题数方面,新加坡教材远多于我国教材。从表 3可知,新数学教材比人教版教材多出1 376道,约是人教版教材的2.18倍。如此大的差异主要由于两国教材章节数和例题与习题模块编排上的不同导致。经比较发现:(1)在章节方面,新数学初中二年级教材比人教版初中二年级教材多出3章内容;(2)在模块设计方面,人教版教材主要分为例题、练习、习题和复习题4个模块,而新数学教材则包括工作示例、课堂练习、习题、复习题、挑战自我和修正复习等6个模块。也就说新数学教材比人教版教材多设置了“挑战自我”和“修正复习”2个模块。
从记忆型题目与理解型题目的占比来看,两国教材都更重视理解型题目。相比之下,新加坡教材对理解型题目的重视程度更高。同时,两国教材并未忽视记忆型题目的练习。两国教材记忆型题目与理解型题目之比都接近4∶6,其中新加坡理解型题目占比超过60%,高出我国3.51%。这在一定程度上表明随着全球数学教育改革的推进,中新两国教材都比较重视学生数学思维的培养。关于记忆型题目,两国教材分别约占40%的比例,这说明基础知识的掌握和基本技能的训练都得到了两国的重视。总的来说,两国教材都在确保基础知识和基本技能训练的前提下,注重提升学生思维与理解能力。
-
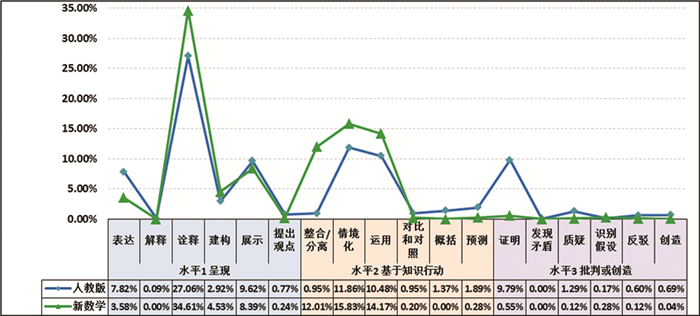
中国、新加坡两国初中数学教材在理解表现各个方面的占比情况如图 1所示。由图 1可看出两国初中二年级数学教材的理解表现具有如下几点共性:(1)均以水平1“呈现”和水平2“基于知识行动”为主,随着理解水平升高,理解表现的占比呈递减趋势;(2)对18种理解表现的重视均呈现不均衡性,且两国教材所重视和弱化的理解表现大多一致。如图 1所示,“诠释”“情境化”和“运用”等理解表现的占比较高,而“解释”“提出观点”“概括”和“创造”则几乎没有涉及;(3)“诠释”“情境化”和“运用”这三项排名前三。其中“诠释”在两国教材中的占比均为第一,这与数学学科的抽象性和概括性密不可分,对数学语言、符号、结构的识别和说明是学好数学的必备能力。
此外,从图中可以看出,两国教材也存在一些差异。两国教材在水平1“呈现”的总体占比比较接近,其中“表达”的占比人教版教材更高,而“诠释”的占比则恰好相反。在“基于知识行动”这一水平上,新加坡教材在各个方面的重视程度都高于我国教材。这可能与新加坡长期以来对数学问题解决能力的重视密不可分。新加坡中小学数学课程的核心目标特别提出要发展学生解决数学问题的能力。新数学教材中独有的“挑战自我”模块多属于水平2的题目,而且该模块多涉及非常规的、开放性的、现实的问题。最后在“评判与创造”水平上,我国教材在各个方面占比都高于新加坡教材。不过明显差异主要体现在“证明”这一个方面,其余方面则相差极小。人教版教材“证明”的占比多出新数学教材约9.2%,表明我国教材对学生逻辑思维能力的高度重视,这可能也受我国长期以来重视证明的影响。在我国,《义务教育数学课程标准(2011年版)》也指出:推理的发展应贯穿整个数学学习过程中[2]。不过,特别要指出的是,人教版教材水平3的题目大多归属于“证明”这一理解表现。除“证明”之外的其他理解表现情况,我国教材的占比同新加坡相似。这表明两国教材对“发现矛盾”“批判”和“创造”等高层次认知能力培养所创设的认知机会极少。
-
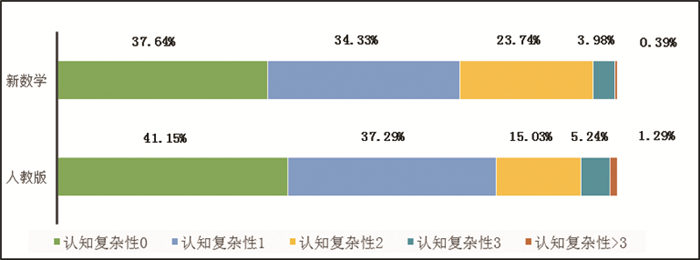
按照前述方法对教材例题、习题的认知复杂性进行赋值,再计算各级题目数量占总题数的百分比,从中可了解和对比中国、新加坡两国初中数学教材例题和习题的认知复杂性(如图 2所示)。
如前所述,复杂性处于0级的题目就是指记忆型题目。通过对比分析中国、新加坡两国初中数学教材中理解型题目的认知复杂性,发现两国教材都更重视培养学生利用单一理解表现或结合两种理解表现解决问题的能力。随着复杂性的增加,两国教材例题和习题的数量都呈现出逐级递减的趋势。应该说,这比较符合学生的认知规律。作为数学学习的初级资料,教材例题、习题是学生掌握知识的首个挑战,由此不宜太过复杂。不过虽然两国教材理解型题目的认知复杂性大多集中在1级或2级,但这两级之间的占比跨度差异较大。人教版教材跨度明显大于新数学教材。新数学教材1级与2级占比相差约10个百分点,而人教版教材则相差近22个百分点。显然,认知复杂性的跨度太大、升级太快无疑会增加学生的学习难度和课业负担。
-
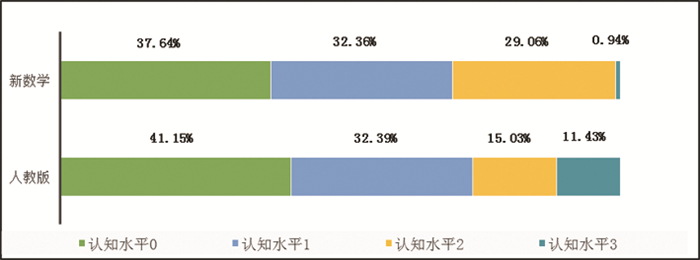
基于对教材例题和习题认知水平的赋值,计算各级水平题目数量占总题数的百分比,以此了解和对比中国、新加坡两国初中数学教材的认知水平(如图 3所示)。
通过对比两国理解型题目的认知水平,可以看出两国教材例题和习题都以水平1和水平2居多,且占比随认知水平升高而下降。同时,人教版教材水平1和水平2占比的跨度远大于新数学教材。新数学教材水平1与水平2占比相差约3个百分点,而人教版教材相差约17个百分点,人教版教材的两级间的跨度几乎是新数学教材的6倍。相较之下,新加坡教材则更能体现“循序渐进”的编排特点。此外,如前所述,由于人教版教材水平3的题目集中于“证明”这一理解表现,而其余理解表现占比与新数学教材相似。
一. 例题和习题数量比较
二. 18种理解表现的占比情况比较
三. 教材认知复杂性比较
四. 教材认知水平比较
-
本研究通过借鉴Harpaz等人提出的“理解表现”框架,系统比较了中国和新加坡两国初中二年级数学教材例题及习题蕴含的认知机会,得出两国教材的异同如下:
-
就题型分布而言,两国教材记忆型题目与理解型题目之比均约为4:6。记忆型题目指向学生双基学习,理解型题目指向思维能力的培养,二者相辅相成。就例题习题总量而言,新加坡总题数约是我国的2.18倍,这主要由于我国教材章节数和模块数少于新加坡教材所致。
-
两国教材对思维能力的侧重上呈现极大的相似性,都重视“诠释”和“情境化”等理解表现,但都含有较少的“批判与创造”等高认知水平的题目(我国教材涉及的高水平例题和习题仅集中在“证明”)。不过教材作为期望课程和实施课程的最关键的中间载体,需要兼顾各种不同思维能力的培养。由此就需要有目的、有计划地进行系统规划。
-
研究结果显示,我国教材“证明”题占比突出,可见我国对学生演绎推理能力训练的重视。然而反观水平2“基于知识行动”,新加坡教材占比则显著高于我国。水平2涉及的问题旨在培养学生解决非常规的、开放性的和现实的数学问题的能力。在数学问题解决能力的培养方面,虽然我国经历了几十年的研究与实践,已经初步形成了自己的一些特色[14],但我国学生缺乏问题解决能力的现状仍不容乐观[15]。教材对培养学生的数学问题解决能力重视不足或许是导致此现状的因素之一。
-
研究结果显示,无论是例题和习题的认知复杂性还是其认知水平,两国教材占比都随等级升高而下降。然而我国数学教材在认知复杂性和认知水平1、2级的跨度远大于新加坡,新加坡教材在此两级占比相当,跨度平缓。
-
数学学习需要相当数量的练习,认知水平的提高亦需要学生进行高认知水平数学任务的实作和练习。教材作为潜在的实施课程,肩负着为学生提供认知机会,发展学生对数学本质的认识的重任,因而教材例题、习题在“量”上首先就应该有必要的保障。研究结果表明我国教材例题和习题数量偏少。对此,可以一方面适当增加例题及习题数量,通过对教材知识点的精细化处理,有针对性地设置相应问题。尤其可以考虑增加一定量的非常规和开放性问题。
另一方面义务教育阶段教材是面向全国学生编写的,但学生的数学认知水平参差不齐,这就需要教材编写者能将学生思维水平的差异性考虑进去,针对不同水平的学生,设计相匹配的模块任务,为不同水平学生提供一个科学合理的模块选择性学习的参考方案,或将能更精准高效地提升各个层次学生的思维能力。
-
虽然当前“问题解决”已经成为我国数学核心素养的基本要点之一[16],但如前所述,与新加坡教材相比,我国教材在“基于知识行动”相关的各个认知水平活动上都占比较小。而从本质上而言,问题解决是一个多层次的认知活动,是一个包含了多个环节的复杂过程,特别是对非常规性、有挑战性的数学问题,学生需要将概念进行重新组合或采取新奇的、独特的观点,做出不同寻常的反应,寻求无单一标准的答案[17]。因此,对于教材而言,就理应保证学生数学学习过程中,能有机会接触相关任务或体验的过程。由此,为培养学生在处理广泛的、不同类型的和新的问题中找到解题的思路,我国教材可增加问题解决一般性原则、程序和策略的内容,根据问题解决的策略组织问题。同时为深化理解,可就一种策略设置多种问题情境进行练习,通过不同例子深入理解这些问题解决的策略,促进有效迁移。
-
如克莱因指出的,“数学研究的推动力不是推理,而是想象力……必须将数学研究看成是一个创造性过程”[18]。这就意味着数学教育目标不能仅仅局限在算法的学习与技能的训练,还应注重数学创造力的培养,而教材例题和习题正是落实学生经历思考、质疑、批判、创造等思维过程的关键载体。内罗克把数学创造力描述为从不同的视角分析问题,产生多元思想,再选择合适的方法去处理不熟悉情境的能力[19]。由此,于教材建设而言,就可以考虑增设开放题、多解性问题或条件不完整等类型的高认知水平问题,增加学生以独创性的、个性化的解决问题的机会。
此外,为了使学生切实经历构思、尝试解决、重新制订计划直至最终解决问题等一系列涉及创造性活动的过程,教材例题及习题可多以设计或具体活动为核心,以这种方式鼓励学生发挥想象力,利用批判性思维对产生出的所有新想法、方案或成果进行评估,选出最佳方案并付诸实践。
-
一般而言,学生的数学认知发展具有阶段性的特征,相应的,学生对数学内容的理解也就具有过程性和层次性等特征[20]。换句话说,学生对数学内容的理解是一个由浅入深的逐渐提升的过程,在此过程中,学生的数学理解也会表现出不同的层次。为了更好地促进和巩固学生对相关数学内容的理解,教材例题、习题的设计和配置过程中就应遵循学生的这种数学学习规律和数学认知发展规律,为处于各个数学理解层次或处于各种理解程度的学生设计和配置一定的量的数学任务。更为重要的是,例题和习题的认知复杂性和认知水平也要有合理的梯度。
然而,如前所述,在例题和习题的认知复杂性和认知水平两个方面,与新加坡教材相比,我国教材跨度都比较大。这种设置虽然在一方面可以保证学生在较低水平数学任务上得到充分的练习。但对于较高水平数学认知发展而言,其练习就显得不够充分。特别是,如前所述,与新加坡教材相比,我国教材在最高认知复杂性和认知水平的数学任务上,都占有一定比例。为了使尽可能多的学生能顺利实现各个层级之间的过渡,就更需要加强中间层次的训练。因此,在后继教材修订中,就有必要重新审视各种认知复杂性和认知水平数学任务的分配。只有更为科学合理地设置数学教材中例题、习题的认知复杂性和认知水平梯度,才能一方面更有效地促进学生数学认知发展,另一方面保证处于各个认知层次的学生都能得到必要的挑战和发展。



 下载:
下载: