-
众所周知,偏微分方程的求解是比较困难的,一般计算的方法都是经过差分将其离散化,进而得到相应的偏差分方程,然后通过计算偏差分方程的解来研究偏微分方程的解,因此偏差分方程的解的研究就显得十分重要[1-4].另外,在土木工程、机械控制等实际问题中存在着各种振动现象[5-8],而描述这些现象的数学模型大多是偏微分方程,因此为了研究工程实际问题的振动行为,有必要对偏差分方程的解的振动特性进行研究.然而在研究偏差分方程的振动特性时,受到Laplace反变换计算难度的影响,偏差分方程的振动性研究成果相对较少[9-12].文献[13]借助包络理论研究了偏差分方程
的振动性,并且给出了方程振动的充要条件.文献[14-16]进一步研究了三类二阶三系数偏差分方程的振动性,同时给出了相应方程解的不同振动条件,本文在文献[14-16]的基础上,考虑一类三阶三系数偏差分方程
的振动性.
HTML
-
为了顺利给出方程(1)的振动条件,首先给出引理1.
引理1[17] 设A,B,C,F是定义在
$\mathbb{R}$ n上的n元可微函数,Γ是Ax+By+Cz=F确定的n参数平面族,Σ是Γ的包络,则Ax+By+Cz=F没有实根当且仅当没有Σ的切平面通过点(x,y,z).相应地,如果设$\widetilde A, \widetilde B, \widetilde F$ 是定义在$\mathbb{R}$ n上的n元可微函数,$\widetilde {\mathit{\Gamma}} $ 是$\widetilde Ax + \widetilde By = \widetilde F$ 确定的n参数直线族,$\widetilde{\Sigma}$ 是$\widetilde {\mathit{\Gamma}} $ 的包络,则$\widetilde Ax + \widetilde By = \widetilde F$ 没有实根当且仅当没有$\widetilde{\Sigma}$ 的切线通过点(x,y).定理1 方程(1)是振动的当且仅当p<0,q<0,
$r < - \frac{2}{3}\left[{{{\left({ - \frac{1}{{3p}}} \right)}^{\frac{1}{2}}} + {{\left({ - \frac{1}{{3q}}} \right)}^{\frac{1}{2}}}} \right]$ .证 由于方程(1)是否振动主要由其特征方程是否有正根来判定,因此首先计算方程(1)的特征方程
进而在
$\mathbb{R}$ 3内寻找特征方程(2)没有正根的区域.对于任意给定的正实数对(λ,μ)∈$\mathbb{R}$ 2,方程(2)都可以在$\mathbb{R}$ 3中确定一个平面,因此可将f(x,y,z,λ,μ)=0视为$\mathbb{R}$ 3上的关于λ,μ的双参数平面族.根据包络的定义,由特征方程(2)所确定的双参数平面族的包络S上点满足
故
则
因此,当x<0,y<0时,有
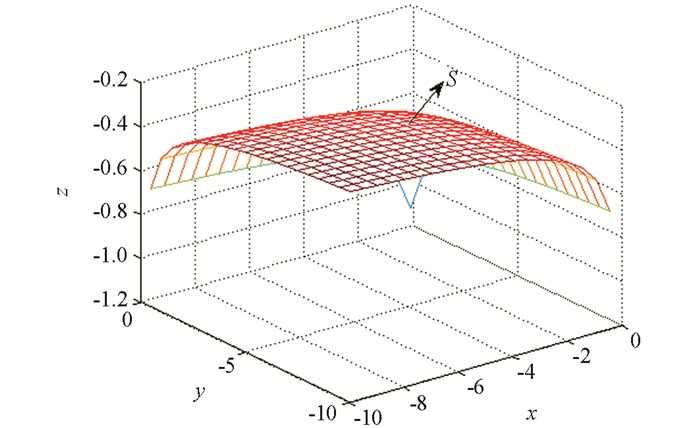
由文献[18]知,当x<0,y<0时,z(x,y)在(-∞,+∞)×(-∞,+∞)上是上凸函数且z(x,y)<0(图 1).
由上凸函数z(x,y)的性质可知,当点(x,y,z)在包络S的下方区域时,不存在包络S的切平面通过点(x,y,z),即当p<0,q<0,
$r < - \frac{2}{3}\left[{{{\left({ - \frac{1}{3}p} \right)}^{\frac{1}{2}}} + {{\left({ - \frac{1}{3}q} \right)}^{\frac{1}{2}}}} \right]$ 时,方程(1)是振动的,同时由引理1可知,该条件为充要条件,故方程(1)是振动的当且仅当p<0,q<0,$r < - \frac{2}{3}\left[{{{\left({ - \frac{1}{{3p}}} \right)}^{\frac{1}{2}}} + {{\left({ - \frac{1}{{3q}}} \right)}^{\frac{1}{2}}}} \right]$ .证毕.
下面给出退化型偏差分方程(1)的振动条件.令特征方程(2)中的一个参数为零,则有
特征方程(5)对应的差分方程变为常差分方程
定理2 方程(6a)是振动的当且仅当p<0,
$r < - \frac{2}{3}{\left({ - \frac{1}{{3p}}} \right)^{\frac{1}{2}}}$ 或者p=0,r≥0;方程(6b)是振动的当且仅当q<0,$r < - \frac{2}{3}{\left({ - \frac{1}{{3q}}} \right)^{\frac{1}{2}}}$ >或者q=0,r≥0.证 方程(6a)的特征方程为
(Ⅰ)当p=0时,特征方程(7)没有正根当且仅当r≥0;
(Ⅱ)当p≠0时,特征方程(7)的系数(p,r)可以看成是
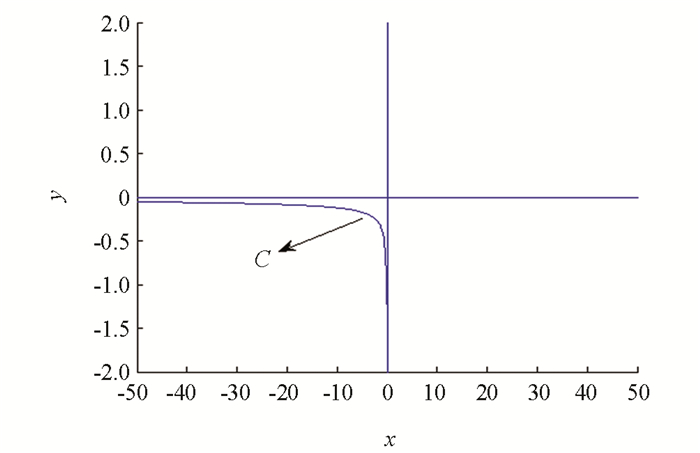
$\mathbb{R}$ 2内的点,只需在$\mathbb{R}$ 2内寻找使得特征方程(7)没有正根的点所在的区域即可.对于任意的正实数λ∈$\mathbb{R}$ ,方程(7)都可以在$\mathbb{R}$ 2中确定一条直线,因此可将f(x,y,λ)=0视为$\mathbb{R}$ 2上的单参数直线族,故由特征方程(7)所确定的单参数直线族的包络C上点满足则
图 2为特征方程(7)所确定的直线族的包络C,且为第三象限中的上凸曲线,当点(p,r)在包络C的下方,即当p<0,
$r < - \frac{2}{3}{\left({ - \frac{1}{{3p}}} \right)^{\frac{1}{2}}}$ 时,方程(6a)是振动的,同时由引理1可知,该条件为充要条件,方程(6a)是振动的当且仅当p<0,$r < - \frac{2}{3}{\left({ - \frac{1}{{3p}}} \right)^{\frac{1}{2}}}$ 或者p=0,r≥0.同理可证方程(6b)是振动的当且仅当q<0,$r < - \frac{2}{3}{\left({ - \frac{1}{{3q}}} \right)^{\frac{1}{2}}}$ 或者q=0,r≥0.证毕.
-
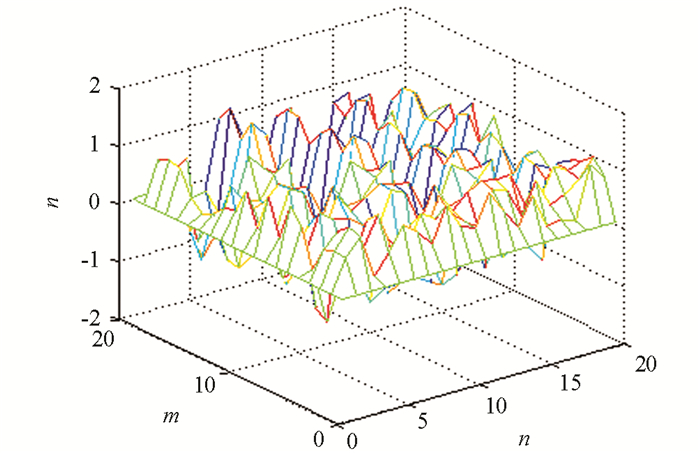
例1 给定偏差分方程
由定理1知,当p=-0.03<0,q=-0.27<0,
$r = - 30 < - \frac{2}{3}\left[{{{\left({\frac{1}{{3 \cdot 0.03}}} \right)}^{\frac{1}{2}}} + {{\left({\frac{1}{{3 \cdot 0.27}}} \right)}^{\frac{1}{2}}}} \right] = - \frac{{80}}{{27}}$ 时,定理1条件满足,因此方程(1)振动(图 3).例2 给定常差分方程
由定理2可知,当p=-2.43<0,
$r = - 0.9 < - \frac{2}{3}{\left({\frac{1}{{3 \cdot 2.43}}} \right)^{\frac{1}{2}}} = - \frac{{20}}{{81}}$ 时,定理2条件满足,因此方程(11)振动(图 4).







 DownLoad:
DownLoad: