-
开放科学(资源服务)标志码(OSID):

-
蚊子是世界上最致命的动物之一,由其传播的疾病每年导致数百万人死亡[1]. 因此,持续的蚊虫控制工作对于预防这些疾病的爆发非常重要.
Wolbachia是一种革兰氏阴性菌,是世界上分布最为广泛的共生菌,并且可以人工植入埃及伊蚊体内[2]. 研究发现Wolbachia可以抑制登革热病毒在蚊子体内的复制并且可以降低病毒的传染性[3],故使用Wolbachia控制蚊媒传染病的传播备受关注. Wolbachia对蚊子的繁殖影响主要有:垂直传播和细胞质分离(CI),即携带Wolbachia的雌性的下一代也会携带此种菌,正常雌性与携带Wolbachia的雄性的下一代会因细胞质分离而死亡.
近年来,许多文献利用数学模型研究了Wolbachia在蚊虫种群中的传播. 文献[4]提出并分析了Wolbachia感染蚊子种群与未感染蚊子种群之间的基本竞争模型,然后,利用反馈控制技术设计了Wolbachia的引入方案. 文献[5-7]分别建立了具有脉冲一般出生和死亡率函数的脉冲模型、具有脉冲出生和投放的性别结构和状态依赖脉冲的综合控制模型,对使用Wolbachia控制蚊媒传染病的各种控制策略进行了研究. 文献[8]考虑了环境的异质性,建立了两种机制随机切换的数学模型. 研究发现:在均匀环境中维持的Wolbachia的初始状态在异质环境中会灭绝,频繁的环境转换有利于Wolbachia的传播. 此外,文献[9-11]等都讨论了宿主、媒介和疾病之间的相互作用.
本文将建立具有成熟时滞的蚊子种群模型,通过理论分析和数值模拟讨论时滞对Wolbachia传播的影响.
全文HTML
-
文献[12]建立了如下数学模型描述Wolbachia在蚊子种群中的传播:
其中:I(t)表示被Wolbachia感染的蚊子数量,U(t)表示未被Wolbachia感染的蚊子数量;β∈[0, 1]是垂直传播的可能性;q∈[0, 1]是CI影响的可能性;b>0是出生率;d>0是死亡率;D≥0是适合度损失. 模型(1)考虑了Wolbachia的垂直传播和CI两种影响,由文献[3]可知,对于某种特定的Wolbachia菌株,其垂直传播率和CI影响发生的可能性都近似接近1,也即,如果雌性蚊子感染了Wolbachia,其后代也会感染;正常雌性蚊子与感染雄性蚊子的后代会因为发生CI而死亡. 所以,我们假设β=1,q=1,并忽略掉适合度的影响(D=0).蚊子的一生有4个阶段(卵、幼虫、蛹、成虫),从卵到成虫大约需要14~21 d,雌性蚊子的寿命约3个月,故蚊子的成熟时间不能被忽略,此因素可用成熟时滞描述. 而成熟时滞又受季节性影响,故考虑为周期时间依赖的时滞,记为τ(t). 根据文献[13]的方法,将τ(t)引入模型(1)中得到如下模型:
其中:δ为幼年蚊子的死亡率,e-δτ(t)是幼年蚊子成熟的可能性. τ(t)是[0,+∞)上的非负、有界且连续可微的ω-周期函数,满足0≤τ(t)≤τ,常数τ=
$\mathop {\sup }\limits_{t \ge 0}$ {τ(t)},τ≥0;μ(t)和ν(t)是关于t∈[-τ,0]的正连续函数.
-
首先引入如下定义:令χ:=C([-τ,0],
$\mathbb{R}^{2}$ ),且具有最大模. 如果函数x(·)∈C([-τ,∞],$\mathbb{R}^{2}$ ),则xt∈χ可被定义为xt(θ)=x(t+θ),∀θ∈[-τ,0]. 对任意ϕ∈χ,定义f(t,ϕ)=(f1(t,ϕ),f2(t,ϕ)),其中因为τ(t)是ω-周期的,故有f(t+ω,ϕ)=f(t,ϕ). 所以,模型(2)是一个ω-周期泛函微分系统.
假设g(t)是一个连续的ω-周期函数,令
则对于系统(2)解的适定性有如下结果.
引理1 对任意ϕ=(ϕ1,ϕ2)∈χ+:=C([-τ,0],
$\mathbb{R}_+^{2}$ ),系统(2)在[0,∞)上有唯一的非负有界解S(t,ϕ)(S0=ϕ).证 在χ+的每个紧子集上,f(t,ϕ)关于ϕ是连续且Lipschitz的. 所以,对任意ϕ∈χ+,系统(2)在其最大存在区间上有唯一的具有初值S0=ϕ的解S(t,ϕ).
定义bc=(1-τ′(t))e-δτ(t)b. 由系统(2)可知:
我们令
则有
易知
又令
并由
可得到
再令
对任意给定的ρ≥1,令
则[0,ρx*]χ是关于χ的有序区间.
容易证明:对任意ψ∈[0,ρx*]χ(ψi(0)=0~(ψi(0)=ρxi*),i=1,2)和t∈
$\mathbb{R}$ ,有fi(t,ψ)≥0~(fi(t,ψ)≤0). 进一步地,[0,ρx*]χ对于系统(2)是正不变的. 通过选择任意大的ρ即可得到解关于χ+的正性和有界性. 证毕.定理1 系统(2)有一个种群灭绝平衡态(0,0),且是不稳定的.
证 (0,0)的存在性容易得到,此略去. 定义Y(t)=(I(t),U(t))为系统(2)的任意解,且|Y(t)|=
$\sqrt{I_{(t)}^{2}+U_{(t)}^{2}}$ . 对于t∈[t0-τ,t0],Y(t)=Φ(t). 定义Φ(t)的范数为||Φ||=sup{|Φ(t)|:t0-τ≤t≤t0}.假设(0,0)是稳定的. 则对任意ε>0,存在δ=δ(t0,ε)>0使得|Y(t)|<ε对于任意的t≥t0和Φ(t)(||Φ||≤δ)成立. 令Y(t)是系统(2)满足I0=inf{I(t):t0-τ≤t≤t0}>0的解,则I0≤δ. 下一步,我们将证明I(t)>
$\frac{I_{0}}{2}$ 对所有的t≥t0成立.假设对任意t≥t0,I(t)>
$\frac{I_{0}}{2}$ 不成立. 则存在一个t1>t0使得对于t∈[t0-τ,t1)有I(t)>$\frac{I_{0}}{2}$ 和I(t1)=$\frac{I_{0}}{2}$ . 所以,I′(t1)≤0. 由系统(2)的第一个方程知:由于|Y(t)|<ε,则有I(t1)<ε和U(t1)<ε. 所以,
我们取
则有
故对任意的t≥t0,有I(t)>
$\frac{I_{0}}{2}$ 成立,矛盾. 所以(0,0)是不稳定的. 证毕.
-
因为τ(t)∈[0,τ],所以,我们首先分析常数时滞的情况. 令τ(t)=r,系统(2)存在如下两个非平凡平衡态(I*,U*):
下分析E1和E2稳定性,将系统(2)在(I*,U*)处线性化后可写为
其中x(t)=(I(t),U(t))T且
这里
且有
假设系统(2)具有形如x(t)=c eλt(c≠0)的解,则可得到系统(3)的特征方程如下:
展开得
其中:a1,a2分别为矩阵A的第一列和第二列;b1,b2分别为矩阵B的第一列和第二列.
可以计算得到
则在E1=(0,
$\frac{\mathrm{e}^{-\delta r} b}{d}$ )处,特征方程为由特征根的符号易知E1是不稳定的.
在E2=(
$\frac{\mathrm{e}^{-\delta r} b}{d}$ ,0)处,特征方程为易知E2是渐近稳定的.
综上可得如下结果:
定理2 在区域{(I,U):I,U≥0}中,当τ(t)=r时,系统(2)有两个非平凡平衡态E1和E2,且E1是不稳定的,E2是局部渐近稳定的.
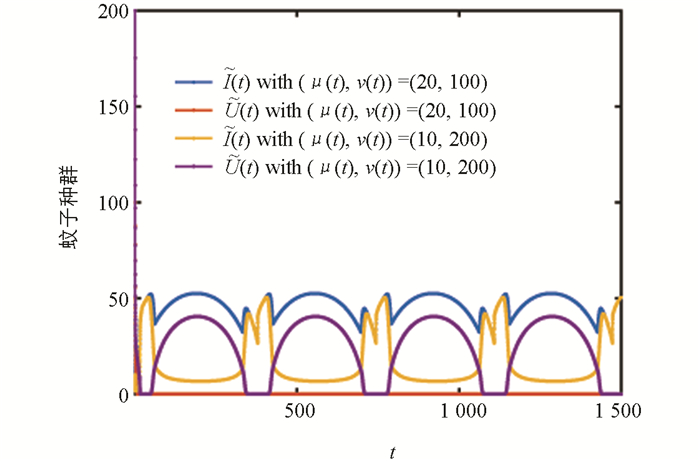
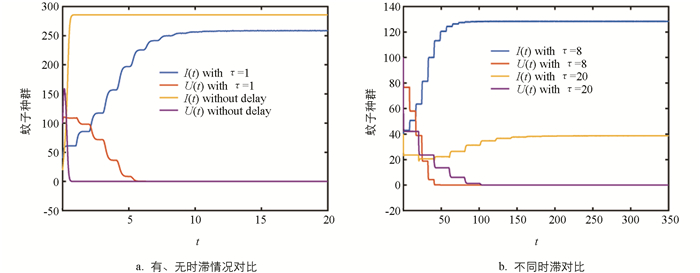
由定理2可知:如果垂直传播是完全的,Wolbachia最终将完全入侵蚊子种群. 图 1a将系统(2)在有时滞((μ(t),ν(t))=(20,100))和无时滞((I0,U0)=(20,100))两种情况下的动力学行为做了比较,比较结果见表 1. 表 1说明:无时滞的ODE系统将高估蚊子总量,这对蚊子种群的控制是不利的,因此成熟时滞对于蚊子种群的动力学行为有重要影响.
图 1b将不同时滞对系统(2)动力学行为的影响做了对比. 可以看出:时滞越小,系统(2)解趋于稳定的时间越短,也即,如果环境有利于蚊子的成熟,Wolbachia完全入侵蚊子种群的速度会加快. 但从蚊子总量看,相比于大时滞,小时滞会使环境中有更多的蚊子. 在实际中,我们应该寻找平衡这两种互反影响的方法.
当成熟时滞是周期函数τ(t)时,我们将通过数值模拟讨论时滞对系统(2)动力学行为的影响. 根据广州市的登革热数据,拟合出成熟时滞表达式为:
其中
并且
图 2显示了不同历史值对系统(2)周期解的影响. 可以看出:(μ(t),ν(t))=(20,100)时,正常蚊子种群将会灭绝;(μ(t),ν(t))=(10,200)时,正常蚊子种群与携带Wolbachia的蚊子种群共存,并呈现周期波动. 从取值来看,当携带Wolbachia的蚊子种群在历史值中所占比例较大时,Wolbachia会完全入侵野生蚊子种群.
图 3在图 2历史值的基础上,研究了不同出生率对系统(2)周期解的影响. 图 3a中(μ(t),ν(t))=(10,200),当b=20时两种蚊子种群共存,当b=40时正常蚊子种群灭绝,图 3b中(μ(t),ν(t))=(20,100),当b=10时两种蚊子种群共存,当b=20时正常蚊子种群灭绝,说明出生率越大,越有利于Wolbachia的传播.
-
本文主要研究成熟时滞对Wolbachia传播的影响. 研究发现:①对于常数时滞,时滞越大越有利于疾病的控制;②携带Wolbachia的蚊子种群在历史值中所占比例越大,越有利于Wolbachia的传播;③蚊子种群出生率越大,越有利于Wolbachia的传播.



 下载:
下载: