Generalizations of Navigation Problems on Riemann Manifolds

CHENG Xin-yue; QU Qiu-hong

doi:10.13718/j.cnki.xdzk.2021.04.011

The navigation problem on Riemannian manifolds plays a very important role in Finsler geometry. Both Randers metric and Kropina metric can be characterized by the solution of the navigation problem on a Riemannian manifold (M, h) with a vector field W satisfying ‖W‖≤1. First, the important relationships between navigation problems and the geometry of indicatrix on Finsler manifolds are revealed in this paper. When the vector field V=V(x) on Finsler manifold (M, Φ) satisfies Φ(x, -V_x) < 1, it is proved that the Finsler metric F determined by navigation data (Φ, V) is a regular Finsler metric; and that when Φ(x, -V_x)=1, F is a conic Finsler metric. Next, the navigation problems on Kropina manifolds and Randers manifolds are studied. When F is a Kropina metric on a manifold M and vector field V satisfies F(x, -V_x)≤1, it is proved that the solution F of the navigation problem with the navigation data (F, V) is either a Randers metric or a Kropina metric. Further, when F is a Randers metric and the vector field V satisfies F(x, -V_x)=1, it is proved that the solution F of the navigation problem with the navigation data (F, V) must be a Kropina metric.

HTML

众所周知，求解Zermelo导航术问题一直是探索和研究芬斯勒空间结构的重要方法之一，对推进芬斯勒几何的研究发挥了重要作用. Zermelo导航术问题是考虑度量空间中的一个运动物体在内力和外力的作用下从一个点运动到另一个点所走的最短时间路径问题^[1-2]. 文献[1]在芬斯勒空间上推广了Zermelo导航术问题，证明了在芬斯勒流形(M，F)上，在满足F(x，-W_x)＜1的外力场W的影响下，最短时间路径是由方程

确定的新的度量F的测地线. 一般地，度量F称为由导航数据(F，W)确定的导航术问题(1)的解. 当F是黎曼度量$h=\sqrt{{{h}_{ij}}{{y}^{i}}{{y}^{j}}}$，且外向量场$W={{W}^{i}}\frac{\partial }{\partial {{x}^{i}}}$满足‖W‖_h＜1时，通过

得到的解为

其中W₀=W_iyⁱ，W_i=h_ijW^j，λ=1-‖W‖_h²＞0. 容易验证‖W_x‖_h=‖β_x‖_α. 此时，F是Randers度量，我们把(h，W)称为Randers度量的导航数据^[1-2].

另外，设$h=\sqrt{{{h}_{ij}}{{y}^{i}}{{y}^{j}}}$是流形M上的黎曼度量，$W={{W}^{i}}\frac{\partial }{\partial {{x}^{i}}}$是流形M上的一个向量场并且满足‖W‖_h=1，那么通过解(2)式可得

此时，F是Kropina度量. 由(3)式确定的度量F在切丛TM上并不是正则的，但在锥形区域A=∪_x∈MA_x上是正则的，这里

我们称这样的度量为锥Kropina度量. 如果令

则由(3)式确定的Kropina度量F也可等价地表示为

此时，F仍然在锥形区域A=∪_x∈MA_x上是正则的，且A_x可表示为

反过来，给定一个Kropina度量$F=\frac{{{\alpha }^{2}}}{\beta }$，令

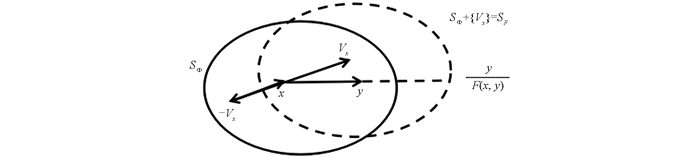
则由(6)式得到的导航数据(h，W) 满足‖W‖_h=1，并且容易验证由导航数据(h，W)确定的(2)式的解正是F. 所以Kropina度量$F=\frac{{{\alpha }^{2}}}{\beta }$与满足‖W‖_h=1的导航数据(h，W)是一一对应的. 我们称(h，W)为Kropina度量F的导航数据^[3].

从本质上讲，芬斯勒流形上的Zermelo导航术问题与流形的单位切球的几何有着密切的关系. 事实上，芬斯勒流形上的导航术问题总对应于单位切球沿一个给定向量场的平移. 具体地，在芬斯勒流形(M，Φ)上，任意点x∈M处的单位切球S_Φ是切空间T_xM在原点x附近的一个封闭超曲面，S_Φ={y∈T_xM：Φ(x，y)=1}. 假定V=V(x)是流形M上的一个向量场，并且Φ(x，-V_x)≤1，考虑S_Φ沿向量场V的平行移动S_Φ+{V}. 易知y∈S_Φ+{V}当且仅当Φ(x，y-V_x)=1，即在切空间T_xM上，S_Φ+{V}的方程为Φ(x，y-V_x)=1. 进一步，我们有以下两个重要的观察结果：

(Ⅰ)当Φ(x，-V_x)＜1时，-V_x的终点在S_Φ的内部，且沿V_x作平行移动后一定与原点x重合. 此时可知S_Φ+{V}包含原点x(图 1).

在这种情况下，易见对于任意的y∈T_xM-0，一定存在唯一正数F=F(x，y)＞0，使得

这等价于F(x，y)满足

可以证明，这样的F=F(x，y)是正则的芬斯勒度量^[3]. 显然，由(8)式可知F是由导航数据(Φ，V)确定的导航术问题的解.

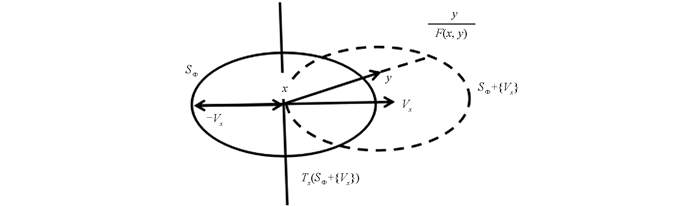
(Ⅱ)当Φ(x，-V_x)=1时，-V_x的终点在S_Φ上，且沿V_x作平行移动后一定与原点x重合. 此时，易见S_Φ+{V}必定通过原点x(图 2).

在这种情况下，易见对于任意的y∈A_x={y∈T_xM：g_V(y，V_x)＞0}，存在唯一正数F=F(x，y)＞0，使得F=F(x，y)满足(7)式，亦即等价地满足(8)式. 此时，F=F(x，y)是锥芬斯勒度量.

上述观察对深刻认识导航术技巧的几何本质，深入推进利用导航术技巧来揭示芬斯勒空间的几何性质与结构的工作是极为重要的.

导航术技巧对于研究芬斯勒几何中的许多问题有着重要的作用，已受到了几何学家们越来越多的关注. 文献[4]利用黎曼流形上的导航术技巧完全刻画和分类了具有常数旗曲率的Randers度量. 进一步，文献[5]刻画和完全分类了具有弱迷向旗曲率的Randers度量和弱Einstein-Randers度量. 文献[6]利用黎曼流形上的导航术技巧刻画和分类了具有常数旗曲率的Kropina度量. 文献[7]进一步决定了具有弱迷向旗曲率的Kropina度量的局部结构. 文献[8]研究了Randers流形(M，F)上的Zermelo导航术问题，证明了：由F和满足F(x，-V_x)＜1的共形向量场V作为导航数据所得到的Zermelo导航术问题的解是一个Randers度量F，并决定了F与F的S-曲率、旗曲率和Ricci曲率性质之间的关系. 文献[9]还研究了由一个Douglas型的Randers度量F与一个满足F(x，-V_x)＜1的向量场V作为导航数据所确定的导航术问题的解F的曲率性质. 另一方面，文献[10]研究了由一个芬斯勒度量F和一个位似向量场V确定的导航术问题的解F的旗曲率性质，并建立了F与F的旗曲率之间的关系，为利用导航术技巧深入研究芬斯勒度量的旗曲率性质提供了新的路径.

本文着重研究Kropina流形与Randers流形上的导航术问题，证明了当F为Kropina度量且向量场V满足F(x，-V_x)≤1时，由导航数据(F，V)确定的导航术问题的解F必然是Randers度量或Kropina度量. 进一步，当F为Randers度量且向量场V满足条件F(x，-V_x)=1时，我们证明了F必然是Kropina度量.

1. 预备知识

在这一节中，我们简要介绍关于Randers度量及Kropina度量的知识.

给定流形M上的黎曼度量$\alpha =\sqrt{{{a}_{ij}}{{y}^{i}}{{y}^{j}}}$和1-形式β=b_i(x)yⁱ，(α，β)-度量是形如

的度量，这里φ=φ(s)是定义在(-b₀，b₀)上的正的光滑函数，且满足

当φ=1+s时，所得到的(α，β)-度量即是Randers度量F=α+β. Randers度量是由物理学家G. Randers在1941年研究广义相对论时引入的，是最简单的一类非黎曼芬斯勒度量. 一个基本的事实是，Randers度量F=α+β正则的充分必要条件是b=‖β‖_α＜1(见文献[2]). Randers度量具有许多重要的曲率性质，关于Randers度量$F=\frac{{{\alpha }^{2}}}{\beta }$的研究也极大地促进了对一般的芬斯勒度量的研究^[11]. 当$\varphi =\frac{1}{s}$时，所得到的(α，β)-度量即是Kropina度量. Kropina度量最初由L. Berwald在讨论二维射影平坦芬斯勒度量时提出，此后引入到热动力学理论等领域. Kropina度量是芬斯勒几何中一类形式简单但极为重要的度量，它在物理学、电子光学、力学等领域中也有着重要的应用^[12-14].

如前所述，Randers度量和Kropina度量都可以通过黎曼流形上的导航术确定. 在流形维数大于2时，Randers度量与Kropina度量都是C-可约的芬斯勒度量^{[12, 15-16]}. 这两类度量在生态学中的Krivan问题的研究中有着重要的应用^[13]. 但是，Randers度量是正则的芬斯勒度量，而Kropina度量是非正则的芬斯勒度量. 具体地，当上述的s在任意区间(-b₀，b₀)上取值时，相应的Kropina度量$F=\frac{{{\alpha }^{2}}}{\beta }$是具有奇异性的芬斯勒度量.

本文着重讨论和研究正则的Kropina度量，把Kropina度量定义在切丛的锥形区域A=∪_x∈MA_x上，其中

显然，此时的Kropina度量$F=\frac{{{\alpha }^{2}}}{\beta }$：A→(0，+∞)为正则的芬斯勒度量. 我们把这样的Kropina度量称为锥Kropina度量^{[3, 6]}. 本文所涉及到的Kropina度量均为锥Kropina度量. 在不引起歧义的情况下，本文总是用术语Kropina度量代替术语锥Kropina度量.

2. Kropina流形上的导航术问题

本节着重讨论Kropina流形上的导航术问题. 假若在Kropina流形(M，F)上给定了向量场V，且V满足F(x，-V_x)≤1. 根据前面的讨论可知：当F(x，-V_x)＜1时，由导航数据(F，V)确定的导航术问题的解F一定是正则的芬斯勒度量；而当F(x，-V_x) = 1时，由导航数据(F，V)确定的导航术问题的解F一定是锥芬斯勒度量. 具体地，一个有趣的问题是：此时的F到底会是一个怎么样的度量呢? 以下定理为我们给出了明确的答案.

定理1 令$F=\frac{{{\alpha }^{2}}}{\beta }$是n维流形M上的Kropina度量，假设V是流形(M，F)上的向量场且-V_x∈A_x，并且满足F(x，-V_x)≤1. 则：

(ⅰ)若F(x，-V_x)＜1，则由导航数据(F，V) 确定的导航术问题的解为

其中W_i=-(2V_i+b_i)，h=2α，λ=-(‖V_x‖_α²+2ρ)，ρ=b_iVⁱ，V_i=a_ijy^j，V₀=V_iyⁱ. 此时，F是正则的Randers度量.

(ⅱ) 若F(x，-V_x)=1，则由导航数据(F，V) 确定的导航术问题的解为

其中W_i=2V_i+b_i，h=α. 此时，F是Kropina度量.

证首先，由-V_x∈A_x可知F(x，-V_x)＞0，即-b_iV_i＞0. 进一步，由方程(1)知，由导航数据(F，V)确定的导航术问题的解F满足

即

把(10)式化简后得

进一步，有

其中V₀=V_iyⁱ，V_i=a_ijy^j. 对(11)式两边同乘${{{\bar{F}}}^{2}}$，我们可得

其中ρ=V(b)=b_iVⁱ. 整理后，有

(ⅰ)若F(x，-V_x)＜1，我们有$0<\frac{\left\| {{V}_{x}} \right\|_{\alpha }^{2}}{-{{b}_{i}}{{V}^{i}}}<1$，从而可得‖V_x‖_α²+ρ＜0.

进一步，由F＞0，可得(13)式的解为

由于$\left\| {{V}_{x}} \right\|_{\alpha }^{2}+\rho <0$，令$\bar{\lambda }=-\left( \left\| {{V}_{x}} \right\|_{\alpha }^{2}+\rho \right)>0$. 再令W_i=-(2V_i+b_i)，h=2α，从而

此时，F是一个Randers度量.

记$\bar{\alpha }=\sqrt{{{{\bar{a}}}_{ij}}(x){{y}^{i}}{{y}^{j}}}$，$\bar{\beta }={{{\bar{b}}}_{i}}{{y}^{i}}$，则由(15)式，有

利用文献[2]中的引理1.1.1，可得

其中h^ij=4a_ij，Wⁱ=a^ijW_j=-a^ij(2V_j+b_j)=-(2Vⁱ+bⁱ). 从而，由(16)式和(17)式可得

由此可知，F是正则的Randers度量.

(ⅱ)若F(x，-V_x)=1，即$\frac{\left\| {{V}_{x}} \right\|_{\alpha }^{2}}{-{{b}_{i}}{{V}^{i}}}=1$，可得$\left\| {{V}_{x}} \right\|_{\alpha }^{2}+\rho =0$. 则(13)式等价于

由此可得

令W_i=2V_i+b_i，h=α，则(18)式可重新表达为

显然，此时F是Kropina度量.

3. Randers流形上的导航术问题

由前面的介绍已知，给定Randers流形(M，F)上的一个向量场V，假若向量场V满足F(x，-V_x)＜1，则由导航数据(F，V)确定的导航术问题的解F必然是Randers度量^[8]. 由此产生的一个有趣的问题是：如果给定的向量场V满足F(x，-V_x)=1，那么由导航数据(F，V)确定的导航术问题的解F会是一个怎样的度量? 根据前面的讨论，此时的F一定是锥芬斯勒度量. 进一步，下面的定理告诉我们，此时的F必然是Kropina度量：

定理2 设F=α+β是n维流形M上的Randers度量，V是流形(M，F)上的向量场且满足F(x，-V_x)=1. 则由导航数据(F，V) 确定的导航术问题的解为

其中W_i=V_i-(1+ρ)b_i，h²=α²-β²，ρ=β(V)=b_iVⁱ. 此时，F是Kropina度量.

证由方程(1)知，由导航数据(F，V) 确定的导航术问题的解F一定满足$F\left( x, \frac{y}{\bar{F}(x, y)}-{{V}_{x}} \right)=1$. 从而，我们有

把(19)式化简后得

由于F(x，-Vx)=1，即$\left\| {{V}_{x}} \right\|_{\alpha }^{2}$-(1+b_iVⁱ)²=0. 令ρ=b_iVⁱ，整理(20)式后，有

由(21)式易得

我们令W_i=V_i-(1+ρ)b_i，h²=α²-β²，则(22)式可表达为

此时，F是Kropina度量.

文献[8]研究了Randers流形上的导航术问题. 给定一个Randers流形(M，F)，证明了：由F和满足F(x，-V_x)＜1的向量场V作为导航数据所得到的Zermelo导航术问题的解是Randers度量F. 结合定理1和定理2的结果，我们可以得到以下结论：设F是流形M上的Randers度量或Kropina度量，V是M上的向量场(当F是Kropina度量时，要求-V_x∈A_x)，则当F(x，-V_x)＜1时，由导航数据(F，V)确定的导航术问题的解必为Randers度量；当F(x，-V_x)=1时，由导航数据(F，V)确定的导航术问题的解必为Kropina度量. 本文的研究成果不仅极大地推广了文献[8]中的结果，更为深化导航术技巧在芬斯勒几何研究中的应用提供了有力的支撑.

Figure (2) Reference (16)

Name
	Name cannot be empty!
E-mail
	Mailbox cannot be empty! Mailbox cannot be empty!
Telephone
	Mobile number cannot be empty! Please enter a valid mobile number!
Title

Content
Verification Code

[1]	SHEN Z M. Finsler Metrics with K=0 and S=0[J]. Canad J Math, 2003, 55(1): 112-132. doi: 10.4153/CJM-2003-005-6 CrossRef Google Scholar
[2]	CHERN S S, SHEN Z M. Riemann-Finsler Geometry[M]. Singapore: World Scientific, 2005. Google Scholar
[3]	CHENG X Y, LI T T, YIN L. The Conformal Vector Fields on Conic Kropina Manifolds Via Navigation Data[J]. J Geom Phys, 2018, 131: 138-146. doi: 10.1016/j.geomphys.2018.05.008 CrossRef Google Scholar
[4]	BAO D, ROBLES C, SHEN Z M. Zermelo Navigation on Riemannian Manifolds[J]. J Differential Geometry, 2004, 66(3): 377-435. Google Scholar
[5]	CHENG X Y, SHEN Z M. Randers Metrics of Scalar Flag Curvature[J]. Journal of the Australian Mathematical Society, 2009, 87(3): 359-370. doi: 10.1017/S1446788709000408 CrossRef Google Scholar
[6]	YOSHIKAWA R, SABAU S V. Kropina Metrics and Zermelo Navigation on Riemannian Manifolds[J]. Geometriae Dedicata, 2014, 171(1): 119-148. doi: 10.1007/s10711-013-9892-8 CrossRef Google Scholar
[7]	XIA Q L. On Kropina Metrics of Scalar Flag Curvature[J]. Diff Geom Appl, 2013, 31(3): 393-404. doi: 10.1016/j.difgeo.2013.03.001 CrossRef Google Scholar
[8]	SHEN Z M, XIA Q L. On Conformal Vector Fields on Randers Manifolds[J]. Science China Mathematics, 2012, 55(9): 1869-1882. doi: 10.1007/s11425-012-4414-y CrossRef Google Scholar
[9]	XIA Q L. On the Flag Curvature of a Class of Randers Metric Generated from the Navigation Problem[J]. J Math Anal Appl, 2013, 397(1): 415-427. doi: 10.1016/j.jmaa.2012.07.035 CrossRef Google Scholar
[10]	ESRA SENGELEN S, SHEN Z M. Randers Metrics of Constant Scalar Curvature[J]. Canadian Mathematical Bulletin, 2013, 56(3): 615-620. doi: 10.4153/CMB-2011-187-1 CrossRef Google Scholar
[11]	CHENG X Y, SHEN Z M. Finsler Geometry-An Approach via Randers Spaces[M]. Beijing: Science Press, 2012. Google Scholar
[12]	MATSUMOTO M. On C-Reducible Finsler Spaces[J]. Tensor N S, 1972, 24: 29-37. Google Scholar
[13]	ANTONELLI P L, INGARDEN R S, MATSUMOTO M. The Theory of Sprays and Finsler Spaces with Applications in Physics and Biology[M]. Dordrecht: Springer Netherlands, 1993. Google Scholar
[14]	程新跃, 李婷婷, 殷丽. 关于射影Ricci曲率的比较定理与共形不变性[J]. 西南大学学报(自然科学版), 2019, 41(2): 52-59. Google Scholar
[15]	CHENG X Y, SHEN Z M. Einstein Finsler Metrics and Killing Vector Fields on Riemannian Manifolds[J]. Science China Mathematics, 2017, 60(1): 83-98. doi: 10.1007/s11425-016-0303-6 CrossRef Google Scholar
[16]	CHENG X Y, YIN L, LI T T. The Conformal Vector Fields on Kropina Manifolds[J]. Differential Geometry and Its Applications, 2018, 56: 344-354. doi: 10.1016/j.difgeo.2017.10.004 CrossRef Google Scholar

Message Board

Generalizations of Navigation Problems on Riemann Manifolds

Abstract

References

Access History

通讯作者: 陈斌, bchen63@163.com

Article Metrics

Access History

Other Articles By Authors